Play Like an Idiot

McCulloch-Pitts: “Purposive behavior depends upon how output affects input which, in turn, depends upon a nervous system whose organization can be treated statistically.”

SEER – McCULLOCH – LACAN

To briefly recap: In his Seminar II, Lacan establishes that the cybernetic era has revealed the shift from the discourse of knowledge to the discourse of the machine. For Lacan, Grey Walter’s cybernetic tortoise – which adapts to the conditions of its environment and seems to apprehend itself in the Other– provides a model for a post-cybernetic intersubjectivity. As the seminar proceeds, Lacan will introduce another cybernetic machine with which humans will make an unexpected partnership.

David Hagelbarger & Claude Shannon’s SEER (SEquence Extrapolating Robot)

The SEER (SEquence Extrapolating Robot) was developed by Claude Shannon & David Hagelbarger at the Bell Labs in the early 1950s. Its aim was to outplay a human in the game of odd or even. In the game the player has to guess if their opponent is holding one or two pennies (or marbles) in their hand. In game theory, odd or even is an example of a “zero-sum two-person” game. It is ostensibly the same as “matching pennies” or “ones and twos”, in which the player has to guess what their opponent has chosen.

To personalize the playing experience the machine was made to resembled a human face with two lights for eyes, a button for the nose and a red toggle switch forming a tongue sticking out. This is in keeping with the playfulness of Shannon’s other machines, particularly the “ultimate machine” (1952), the only purpose of which was to switch itself off.

Over the years both Claude Shannon and David Hagelbarger worked on a variety of second guessing machines with various names, including the “Mind-Reading (?) Machine” and the “Outguessing Machine”. SEER (SEquence Extrapolating Robot) was the title considered most scientifically respectable.

Operating diagram of Claude Shannon's Mind Reading (?) Machine

David Hagelbarger (who built the first version of the outguessing machine), with his device.

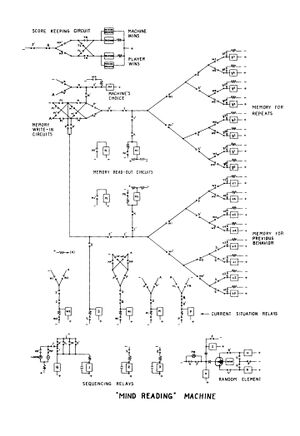
Underlining the machine's playful appearance and function were serious reasons to develop predictive machines: “We hope,” wrote Shannon, “that research in the design of game-playing machines will lead to insights in the manner of operation of the human brain”. Shannon noted at the time, as did Lacan and Guilbaud, that the game of matching pennies had already been used to model human predictive behavior in John Von Neumann and Oskar Morgenstern’s Theory of Games and Economic Behavior and that the game had been a key figure in Edgar Allan Poe’s Purloined Letter. Shannon & Hagelbarger also wanted to develop a machine that could readjust itself and adapt, as Hagelbarger reflected soon after: “Perhaps in an extremely complicated situation it might be easier to design a machine which learns to be efficient than to design an efficient machine as such.” It was also useful, within the communications industry, to have machines that could execute complex actions with humans and other machines whilst using minimum processing power. In the case of SEER the processing power was minimal and yet it was able to produce complex behaviour in both human and machine. The machine had only 16 bits of storage (2 bytes) but was nevertheless able, in a game of long duration, to outguess its human opponent 65%-75% of the time. SEER gave a binary choice between “left” or “right”, in the first move the machine guessed randomly, after which it “learned” from the patterns produced by its opponent. Its choice of left or right would be dependent on the human opponent’s previous choice (its dossier of choices) and on a random choice.

The machine broke the penny-matching model down into eight scenarios, each with a particular probable outcome. For example: if the human player wins twice in a row with the same choice – let’s say “right” –, the human tendency would be to switch on the third move – to “left” –, because to stick with “right” would not be random. Such decisions were recorded as a “1” or “0” in SEER’s 16-bit memory. For each of the eight scenarios the machine recorded the last two only – filling the 16-bit memory. To predict its opponent’s move the machine would check the last two moves taken when the particular scenario in question occurred and choose the same. If the human opponent chose differently it would use a spinning commutator, which gave a random result.

The human was likely to lose against SEER in the majority of cases because it was close to impossible for humans to counterfeit random choice. Shannon, however, notes that he was able to outguess SEER by getting into the “mind” of the machine, mentally emulating the machine’s operation and then doing the opposite. However, because the machine plays randomly, even a perfect human emulator could only ever win 75 % of the time.

In the later stages of the experiment the human factor was eliminated altogether, as Shannon & Hagelbarger had two outguessing machines play each other, with play invigilated by an “umpire” machine.

In March 1953 Claude Shannon wrote a Bell Laboratories memorandum entitled “A Mind-Reading (?) Machine” in which he noted that “[The game of matching pennies/ones and twos had been] discussed from the game theoretical point of view by von Neumann & Morgenstern and from a psychological point of view by Edgar Allan Poe in his story The Purloined Letter." Oddly enough, the machine is aimed at Poe’s method rather than von Neumann’s. In Poe’s story, the detective, Dupin, discusses the game of odd and even on the basis that people can be predicted even when they try not to be. Von Neumann & Morgenstern’s emphasis was on the role of random choice had on the player of the two-person sum-zero game. Lacan’s seminar and lecture addressed the complexity of both these positions. In Theory of Games and Economic Behavior (1944) Von Neumann & Morgenstern had proved mathematically that random choice becomes a defensive position if the two protagonists are evenly matched. If they are evenly matched the introduction of randomness will give the player acting randomly advantage. The machine (SEER) can, however, produce a true random result, which a human opponent to the machine can less readily do.

A source of amusement at the Bell Labs for Shannon & Hagelbarger was that the elite scientists who played with SEER understood it as a test of intelligence. It is the unconscious (non-conscious) structure of such a machine that renders it unbeatable. It was Lacan’s task to stress the importance of this to the participants in his second seminar.

We will see shortly that Lacan’s discourse on cybernetics continues with consideration of Von Neuman & Morgenstern’s s game theory and that the series of +s , –s , 0s and 1s were, for Lacan, units of probability which establish the syntax within a symbolic system.

From the early 1940s Von Neuman had been keen to establish a relationship between the way a computer operates and the way the organic neural system operates. Von Neuman posited that a form of machine intelligence could be achieved if the machine was built on the principles of McCulloch-Pitts model of neuron activity. McCulloch-Pitts became the bridge between a cybernetic approach (which recognised a relation between the operations of servomechanisms and biological systems) and information theory, (which concentrated on the effectiveness of the message and the operations of “thinking machines”).

McCULLOCH – SEER– GOVERNOR–BRAIN

UNIVERSAL TURING MACHINE

Cybernetician Warren McCulloch

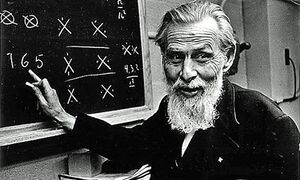
For some years, Warren McCulloch had been arguing that the activity of the human brain could be reproduced in a machine. With John Von Neumann, McCulloch supported the idea that a “logic machine” could be built which exhibited the cognitive abilities of “perception, thought, memory, concept formation, knowledge of universals and their recognition, will, and even consciousness”.

In Ideas Immanent in Nervous Activity (1943), McCulloch and Warren Pitts had put forward the notion that neural activity was produced within a network of circuits, cycles, and loops which are ordered by the laws of probability. Earlier, in 1930, Lawrence Kubie – then a neurophysiologist, before entering a career as a psychoanalyst – had proposed that neurons behave in such a fashion; In 1938 Lorente de No demonstrated experimentally the existence of such cycles in the nervous system. McCulloch and Pitts paper, Ideas Immanent in Nervous Activity mapped out this cycling activity in mathematical, probabilistic terms (building on the logical operations of Leibnitz and Boole). They suggested that single neurones (which in binary action, fire or do not fire) conduct logical operations and act as logic gates which organise information within a nerve net in the brain. In this way, the brain organises a superabundance of information that at any given time showers through the nervous system. Thus the neural net selects and feeds back information which is conducive to adaptation and self-correction.

In 1941 Kubie, now a psychoanalyst, posited that this cycling activity could be the basis for neurosis. This is made possible because as these “reverberating circuits” allow the sequences of events that reach the nervous system to be preserved in memory, “captured and placed beyond the reach of time”. Neurosis would be the cycling of such memories. This cybernetic reading of compulsion (or automatism) was well known to Lacan. You may remember that Freud's term “Wiederholungszwang” was translated by Lacan as “automatisme de répétition” (“automation of repetition”). As the seminar progressed Lacan would draw the distinction between machine “memory” and human “remembering”.

For McCulloch, because neural activity could be formally expressed as code, it followed that the principles of brain function could be applied to model a machine with consciousness. For John Von Neumann this was a quantitive issue rather than a qualitative one –in time the number of switches in a machine would match the number neurons in a human brain. This was also the emphasis of psychiatrists attending the Macy conferences on Cybernetics, including Ross Ashby and Grey Walter.

At the beginning of the 1950s, we were still some distance from a “thinking machine”. The technical standard of the day was set by machines like SEER. In Warren S. McCulloch’s Machines That Think and Want (1950), McCulloch outlines the precise relation between a rudimentary 16-bit computer (such as SEER) and the human neural system. McCulloch proposes that we think of a neuron as “a telegraphic relay system which, tripped by a signal, emits another signal” this takes a millisecond, and again in line with a single state computer, “its signal is a briefer electrical impulse whose effect depends only on conditions where it ends, not where it begins. One signal or several may trip a relay, and one may prevent another from so doing.” McCulloch invites us to understand such signals as the atoms of a molecular event. “Each goes or does not go” (an all-or-none impulse). It can only signal that it was tripped, and its signal implies that it was tripped. “The signal received is an atomic proposition. It is the least event that can be true or false.” McCulloch next introduces a simple symbolic system to map these “atomic units”. The system is comprised of a series of Xs denoting a specific operation.

.X = A happens (a single neuron has been tripped)
X. = B happens
‘X = both happen
X, = neither happen
X = The signal of a neuron never tripped (contradiction).

The system describes several things.

1. the operations of a 16-bit computer (such as SEER)
2. the activities of neurons as units of probability
3. revealing 1. and 2. as expressions of negative entropy

“Every next atomic event, depending on two present atomic events, is shown as a dotted X of which there are 16.” The calculus of propositions in such a scheme is 16. This is essentially the structure of SEER. The operation of each atomic event is linked to probability. If in a particular millisecond we are given ‘X , since its probability is ¼ its amount is 2 units [of information]” Any machine yet built cannot match the number of operations undertaken by the human brain. The human brain is showered with a superabundance of stimuli which mostly fail to coincide. “Information measures the order of the ensemble in the same sense that entropy measures its chaos. In Wiener’s phrase, information is negative entropy; and our X’s the more the dots the greater the entropy.”

An elaboration of the scheme makes this clearer

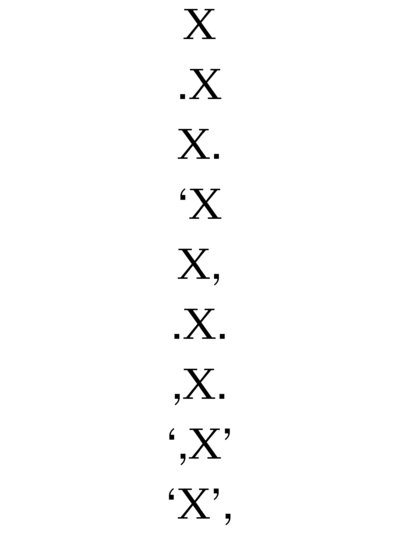
I add this sequence in order to establish that the dotting of the Xs establishes a record. This record represents a “memory”. This “memory” is of a different order to “remembering” a particular event from childhood, for instance, which is subjective. The “memory” here is exterior to the subject, it exists irrespective of the existence of any particular human subject, and yet it makes a claim on human consciousness. This distinction between “memory” and “remembering” will be key to Lacan's reading of cybernetics in Seminar II. Lacan will stress that procedures such as this, embodied in machines such as SEER, make a claim on the human subject which produces the cybernetic unconscious.

In Machines That Think and Want, McCulloch relates his system of Xs and dots to the neural shower, outlining the stochastic nature of thought: “We reject a great deal more information than we receive; we see an agreement occurs in those instances where dots are absent” In this way information is adjustive and produces a filter which consolidates particular atomic events (in this case ‘x or x’ &c) “Circuits with negative feedback return a system toward an established state which is the end of the operation – its goal. Reflexes left to their own devices are thus homeostatic.”

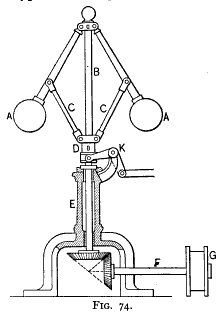
McCulloch next moves to establish a relation between homeostasis within the body and brain, and the governor of the steam engine. Just as each receptor within the body serves to return a given perimeter to its established value, so within the brain the impulses through the thalamus to the cortex are inhibited through limits established by negative feedback. The response of the circuits passing through this system “depends on the time the returning meet the incoming signals”.

Some cycles resonate and oscillate, which may tend toward excitability and instability (positive feedback). Others regulate any such excitation, which results in homeostasis (negative feedback). The servomechanism most importantly operates on the information passing through the system regulated by negentropy, which guards against overrun or positive feedback.

The key point here, particularly as we go on to discuss Lacan’s Seminar II in greater detail, is that McCulloch seeks to establish an equivalence between the operations of a finite state computer, the operations of a servo mechanism, and the operations of the human neural system. Lacan’s seminar was open to discussing the implications of this equivalence, incorporating Von Neumann & Morgenstern's Game Theory and Shannon's Information Theory to bind these connections.

As was discussed in a previous chapter, McCulloch had very little time for the discipline of psychoanalysis, and, after establishing how neural activity (be it man-made or begotten) is organised, next makes a link to purpose.

McCulloch charts how the cycles which produce homeostasis in an organism also produce what we recognise as psychic activity.

“[P]urposive acts cease when they reach their ends. Only negative feedback so behaves and only it can set the link of the governor to any purpose. By it we enjoy appetites which, like records that extend memories, pass out of the body through the world and returning stop the initial eddies that sent them forth.”

This process of what McCulloch terms “apititation” is manifold and may, like the involuntary actions of swallowing and breathing, be contradictory or “incommensurate”. The outcome of such conflict can be described as choice. In this way McCulloch links the operations of the governor to the operations of the psyche, suggesting an alternative to Freudian psychoanalysis (in which motivation is regulated by “psychic energy”). In McCulloch the circuit of “apititation” (desire?) is that of information; which links involuntary actions, such as breathing and swallowing, which are regulated by the same homeostatic circuits. It is the cybernetic discourse of the psyche as a circuit of communication that Lacan addresses in Seminar II. In the next chapter we will examine how Lacan engaged with the cybernetic discourse of probability and feedback in relation to the “second guessing machine”.

The Tortoise and Homeostasis ←

→ Odd or Even?